From the Table of Information, the rotational inertia of a uniform disk with mass
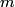 and radius and radius  about its center of mass is about its center of mass is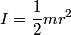 The total kinetic energy of an object is the sum of the kinetic energy of the center of mass and the kinetic energy about the center of mass, so before the disk reaches the ramp, its total kinetic energy is 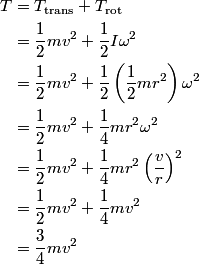 We now set this equal to the gravitational potential energy when the center of mass of the disk reaches its maximum height 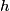 : :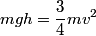 From this, we can solve to find  , ,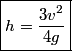 Therefore, answer (B) is correct. |